Make mental maths a breeze with these quick and easy tricks.

Author
Taylor Hartley

Expert Reviewer
Jill Padfield
Published: July 2024


Make mental maths a breeze with these quick and easy tricks.

Author
Taylor Hartley

Expert Reviewer
Jill Padfield
Published: July 2024




Make mental maths a breeze with these quick and easy tricks.

Author
Taylor Hartley

Expert Reviewer
Jill Padfield
Published: July 2024


Key takeaways
Whether going about your daily routine or practicing maths problems after your latest lesson, you will likely use maths every single day. And while this practice can be fun and rewarding on its own, sometimes you need to cut down on time or simply knock out basic maths problems on the way to something more complex.
Fortunately, there are plenty of maths tricks you can learn to save you time and frustration. Memorising a few of these will make your adventures through maths much smoother, and they may even help you and your learner understand more complex concepts down the line.
People struggle with addition when the numbers are not nice and even. For example, adding 73 and 19 might take even the quickest maths minds a second to calculate. For young learners, this can become overwhelming and discouraging.
To make this kind of addition easier, break the numbers down into more manageable chunks.
Let’s use the example above: 73 + 19.
First, take both numbers and break them up.
73 = 70 + 3
19 = 10 + 9
With this approach, you have numbers that are much easier to add.
70 + 10 = 80
3 + 9 = 12
Now, add the two sums together:
80 + 12 = 92
You have your answer, and it was much easier to do in your head.
Another way to take complex numbers and find their sum easily is to round them up to the nearest number ending in zero. For instance, if you wanted to add 347 and 559 together, that might take a second. Instead, try rounding.
First, round each number up to the nearest tenth place:
347 becomes 350
559 becomes 560
Now, you have 350 and 560, which are easier to add together:
350 + 560 = 910
Next, look at how much you rounded each number up and add the total together:
350 – 347 = 3
560 – 559 = 1
3 + 1 = 4
Then, subtract that total from 910:
910 – 4 = 906
That means 347 + 559 = 906.
Unlock unlimited maths questions
Put your skills to the test with fun exercises + maths games that are proven to boost ability!
There are subtraction tricks similar to the addition tricks that will make your daily mental maths a breeze.
To quickly subtract two numbers, you can add enough to one number to make it a multiple of 10 and then subtract. You will then subtract what you added from that number to get your final answer.
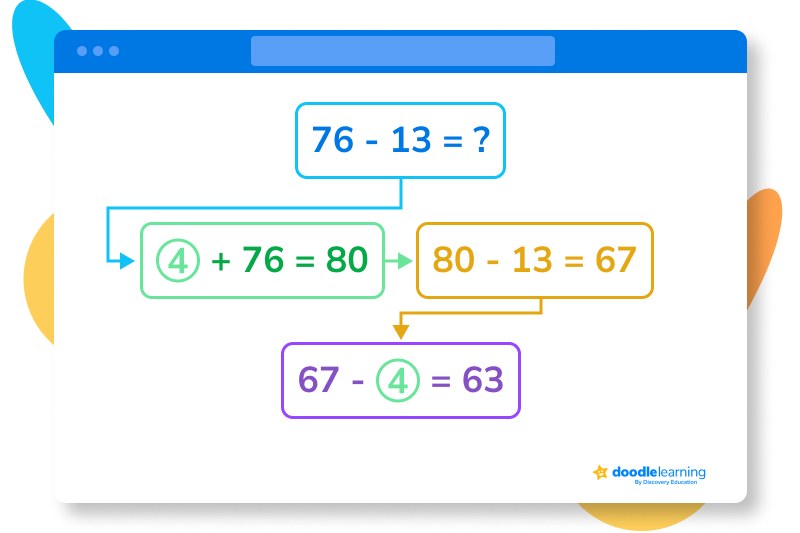
For example, what is 76 – 13 = ?
First, add 4 to 76 to get 80, which is easier to work with.
Now rewrite the problem using 80:
80 – 13 = ?
Subtract 13 from 80:
80 – 13 = 67
Subtract the 4 you added earlier, and you have your answer:
67 – 4 = 63
Therefore, 76 – 13 = 63.
This trick works for addition as well. If you have a problem where the number you are subtracting from is a multiple of the number you are subtracting, you can simply think back to a multiplication chart.
For example, if you have 81 – 9, you know that 81 is a multiple of 9: 9 x 9 to be exact. That means if you subtract 9 from 81, you are looking for the product of 9 x 8, which is 72.
Try DoodleMaths for free!
Select a year group
Depending on the numbers you are multiplying, you will find lots of tricks at your disposal to make multiplication much easier to do in your head.
One way to handle any larger numbers in multiplication is to use their distance from 100. This will work for any two digit numbers.
Consider the problem, 91 x 97.
The first step is to subtract each number from 100.
100 – 91 = 9
100 – 97 = 3
Now, find the sum of those two results:
9 + 3 = 12
Next, subtract that from 100:
100 – 12 = 88
This gives you the first portion of your answer, in the hundreds. So here, you have 8,800.
Now, you multiply the two numbers you got before from the difference from 100:
9 x 3 = 27
Add the product to the first part of your answer:
8,800 + 27 = 8,827
Therefore, 91 x 97 = 8,827.
This works best with double digits numbers that are close to 100, preferably in the 90s, so the addition at the end is easy.
Whenever you multiply an even number by 6, you can find the ones place of the product very easily. The rule of 6 is that the ones place of the product will always match the ones place of the number you are multiplying by 6.
For example, look at 6 x 14. Thanks to this rule, we know the answer will have a 4 in the ones place.
6 x 14 = 84
As you can see, 14 and 84 both have a 4 in the ones place.
You probably already know that all multiples of 5 end in either 5 or 0, but this trick will make finding these multiples even easier.
For any even number you are multiplying by 5, divide it by 2. Add a zero to that number, and you have your answer. Check out 5 x 8.
Half of 8 is 4, and now you can just add a 0 after the 4 to get your answer.
5 x 8 = 40.
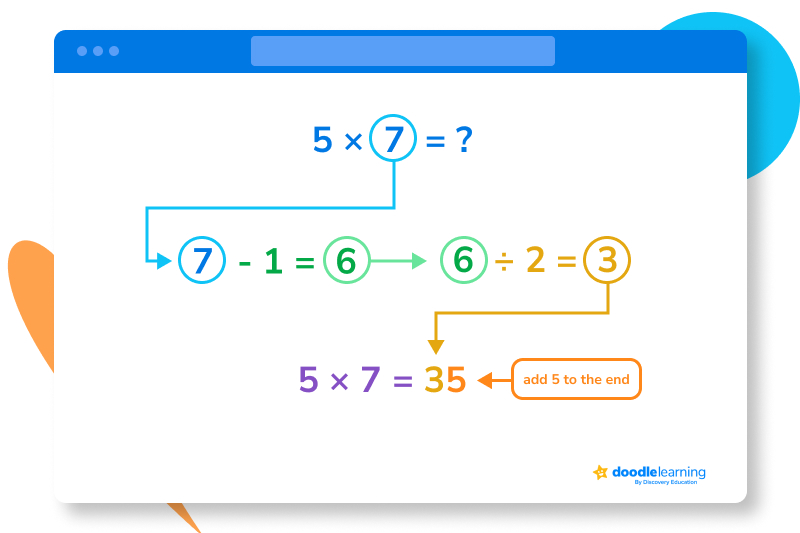
Multiplying 5 by odd numbers is just a little bit tricker, but this maths tip still makes the multiplication a breeze.
Say you want to find the product of 5 x 7. The first step is to subtract 1 from the odd number you are multiplying by 5. That means 7 becomes 6.
After that, just like before, divide this number (6) by 2. This will get 3.
Now, add a 5 after the 3 to get 35. This is your answer.
5 x 7 = 35
If you are trying to remember the multiples of 9, this trick is invaluable. If a number is a multiple of 9, the sum of the individual digits will always equal 9.
For example, 9 x 2 = 18, and 1 + 8 = 9.
Let’s try it with some larger numbers. 9 X 1,223 = 11,007, and 1 + 1 + 0 + 0 + 7 = 9.
If you’re ever unsure about your work, you can use this trick to check your answer.
Adding with your fingers is easy, but did you know you can use those digits to multiply, too? This handy trick allows you to easily figure out multiples of 9. Let’s try this with 9 x 6.
Start with both hands palms out in front of you, all fingers and thumbs extended. For any number you are multiplying by 9 (which is 6 for this example) start counting fingers from the very most left finger. Once you get to 6, put that finger down. For 6, that would be the thumb on your right hand. You should have five fingers up on your left hand and 4 on your right.
To figure out the answer to 9 X 6 from this, look at the number of fingers on both sides of the finger you put down. You have 5 on the left, and 4 on the right. That means your answer is 54.
Multiplying any one-digit number by 11 is a simple trick most people already know: you just duplicate the number!
4 x 11 = 44
7 x 11 = 77
That’s nice and easy. However, multiplying two-digit numbers by 11 can be a little trickier, but it’s still easy with this technique.
All you need to do is add up the two numbers of the multiplicand and place the sum in between the two numbers.
For instance, take 34 x 11.
First, sum the two digits of the multiplicand (34):
3 + 4 = 7
Next, place the sum in between the two digits you multiplied:
Place 7 in between 3 and 4 to get 374
Therefore, 34 x 11 = 374.
If the sum of the first and second digit is greater than 9, still put the sum in the tens place, but add the tens portion to the hundredths place.
For example, 11 x 76 = 836.
The way we got here was by adding the two digits of the multiplicand:
7 + 6 = 13
Since 13 > 9, if you place the 13 in the middle of 7 and 6 you would get 7,136. Instead, add the 7 and 1 together in the hundredths place to get 836.
DoodleMaths is an award-winning app that’s filled with thousands of questions and games exploring multiplication, division and more!
Designed by teachers, it creates each child a unique work programme tailored to their needs, doubling their progression with just 10 minutes of use a day. Try it for free!


Division can be tricky, but, with a few tricks of your own, you can make some basic division completely worry-free.
You can easily determine if a number is divisible by certain other numbers just by looking at the last digit or by doing some simple addition.
Division can be tricky, but, with a few tricks of your own, you can make some basic division completely worry-free.
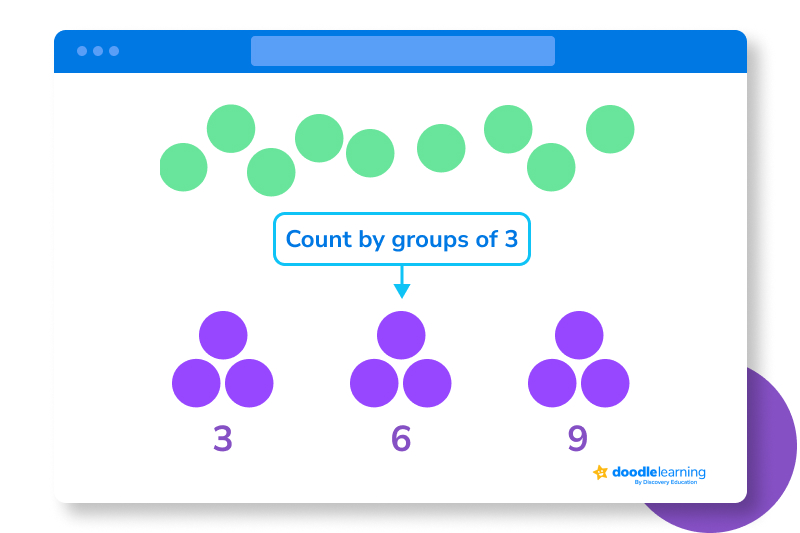
If you have a large quantity of things to count, like pieces of Halloween candy, counting each individual Snickers bar will take a while. Instead, if you count by groups of 2 or 3, you can count much quicker.
Instead of counting 1, 2, 3, and so on, you can count 3, 6, 9 until you are done. This is much faster and also helps you practise with multiples.
You can blow some minds by showing how you can get 1,089 as an answer for some simple maths problems with any three-digit number.
The first step is to take any three-digit number and rearrange the digits into descending and ascending order. For example, take the number 724:
Descending order: 742
Ascending order: 247
Now, subtract the ascending version from the descending version:
742 – 247 = 495
After you have this number, reverse it:
495 reversed is 594
Then, add these two numbers up:
495 + 594 = 1,089
No matter what three-digit number you use for this trick, you will always end up with 1,089.
If you are trying to figure out which of two fractions is the largest one, you can do some quick multiplication to get your answer.
For example, which is larger, 7⁄9 or 8⁄11?
First, you multiply the numerator of the first number by the denominator of the second:
7 x 11 = 77
Now, you multiply the denominator of the first number by the numerator of the second number:
9 x 8 = 72
If the first product is larger, the first fraction is the largest, and if the second product is the biggest, then the second fraction is the largest.
77 > 72
This means 7⁄9 is greater than 8⁄11.
We understand that diving into new information can sometimes be overwhelming, and questions often arise. That’s why we’ve meticulously crafted these FAQs, based on real questions from students and parents. We’ve got you covered!
Some popular tricks include the “Finger Trick,” where using fingers helps quickly determine multiples of nine. Additionally, teaching learners the “Counting by Multiples Trick” allows them to count larger quantities easily while teaching multiples. These tricks not only boost mathematical abilities, but they also instill confidence in young learners.
Mastering these shortcuts can improve problem-solving skills, which helps with maths assignments and real-world challenges. In everyday life, these tricks can help kids quickly calculate tips at restaurants, mentally estimate costs while shopping, or figure out time-related problems effortlessly.
For primary school students, simple maths tricks work best. Learning about patterns in multiples of 10 or 5 is an easy start, and the “rule of 9s” trick can make that part of learning multiplication much easier.
You can introduce maths tricks through interactive games, puzzles, demonstrations, and activities. For instance, organising a “Maths Magic Show” where kids perform tricks for each other fosters a collaborative and engaging learning environment, while making maths fun.


Parents, sign up for a DoodleMaths subscription and see your child become a maths wizard!

Lesson credits

Taylor Hartley
Taylor Hartley is a freelance writer. When she's not writing, you can find her on the rowing machine or lost in a good novel.

Jill Padfield
Jill Padfield has 7 years of experience teaching mathematics, ranging from Algebra 1 to AP Calculus. She is currently working as a Business Analyst, working to improve services for Veterans while earning a masters degree in business administration.

Taylor Hartley
Taylor Hartley is a freelance writer. When she's not writing, you can find her on the rowing machine or lost in a good novel.

Jill Padfield
Jill Padfield has 7 years of experience teaching mathematics, ranging from Algebra 1 to AP Calculus. She is currently working as a Business Analyst, working to improve services for Veterans while earning a masters degree in business administration.
Book a chat with our team
If you’d like to use Doodle’s browser version, please visit this page on a desktop.
To log in to Doodle on this device, you can do so through our apps. You can find out how to download them here: