

Discover the power of multiplication and its fundamental properties, making multiplication operations easier to understand and solve.

Author
Michelle Griczika
Published
February 2024


Discover the power of multiplication and its fundamental properties, making multiplication operations easier to understand and solve.

Author
Michelle Griczika
Published
February 2024


Discover the power of multiplication and its fundamental properties, making multiplication operations easier to understand and solve.

Author
Michelle Griczika
Published
February 2024


Key takeaways
Table of contents
Let’s make maths simple. Some special rules, or “properties,” always apply when multiplying numbers. Understanding these multiplication properties makes calculations easier and faster. In this article, we will walk through the five properties of multiplication and practise applying them so you feel confident when tackling any multiplication problem.
The associative property of multiplication is about how we group numbers in a multiplication problem. This property suggests that how we group numbers doesn’t influence the outcome, aka product.
Take 2 x 3 x 4, for example. If you multiply 2 and 3 first and then multiply the result by 4, or multiply 3 and 4 first, then multiply the result by 2, the final product will be the same. As a result, the associative property can simplify complex multiplication problems by allowing us to conveniently group numbers.
The commutative property of multiplication states that the order of numbers in a multiplication problem doesn’t change the result. So, 7 x 3 = 3 x 7.
This property is beneficial when solving problems because it allows us to rearrange numbers to make calculations easier. For example, when solving a multiplication problem involving numerous numbers, you can rearrange the numbers to multiply familiar combinations. This helps simplify the overall calculation.
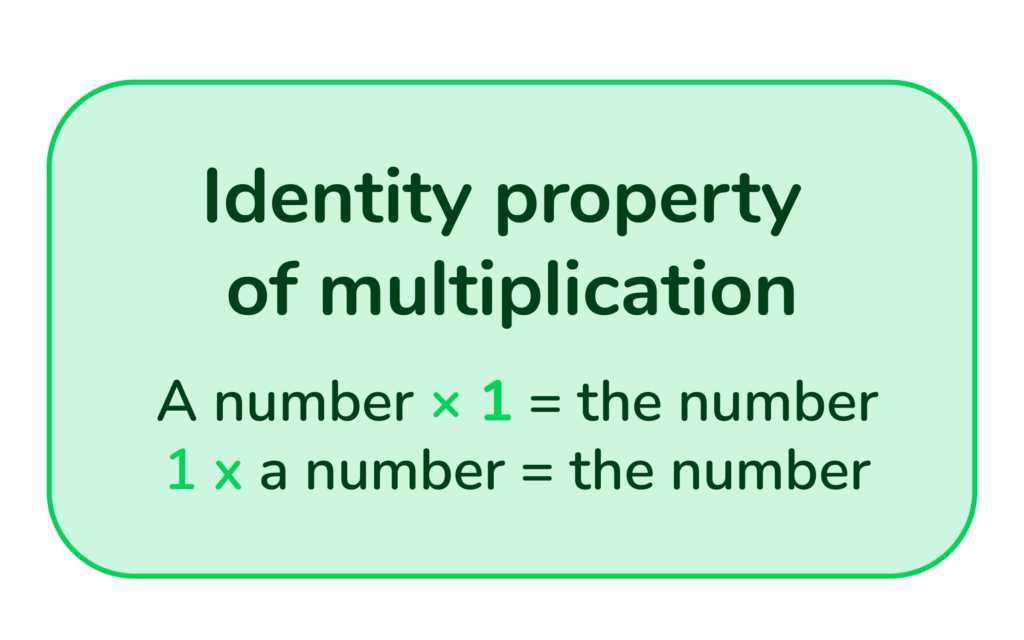
The identity property of multiplication states that a number retains its original value when multiplied by one. Any number multiplied by one will remain the same. For example, when we multiply 5 by 1, the outcome is still 5.
This is true of every number. The multiplicative identity property is crucial because it reminds us that multiplying by 1 doesn’t change the value of a number. It comes in handy when dealing with more complex mathematical equations in alegebra where 1 is often used to preserve the identity of a variable.
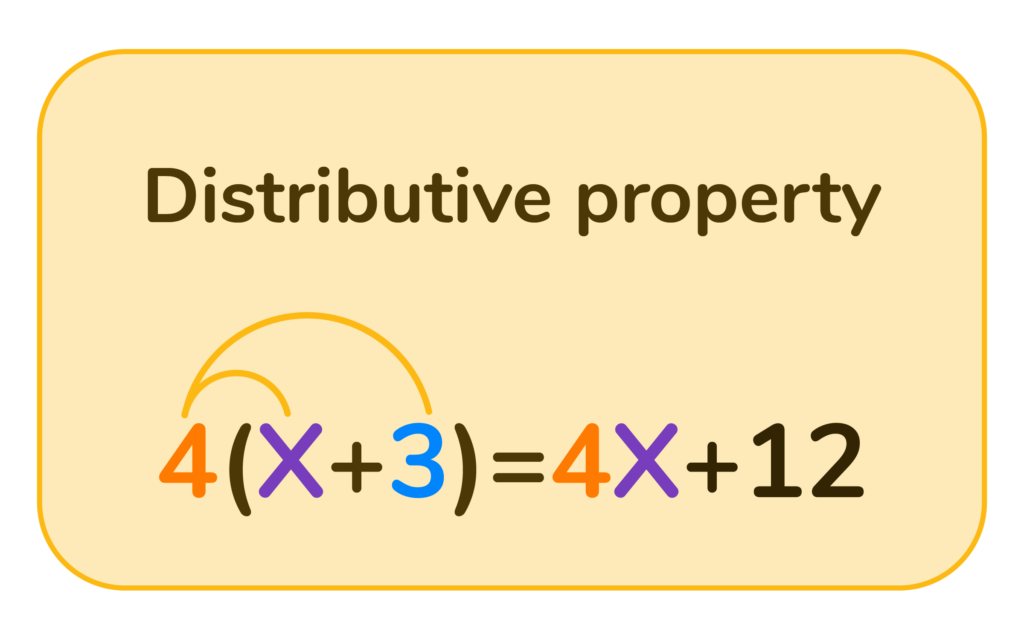
The distributive property of multiplication states that a number multiplied by the sum of two others, like 2 x (3 + 4), is equivalent to multiplying the number by each of the other two separately and then adding those results, as in (2 x 3) + (2 x 4).
This property is especially beneficial for simplifying intricate problems into smaller, more solvable pieces. It’s also the basis for many strategies used in mental maths, allowing us to multiply larger numbers quickly in our heads.
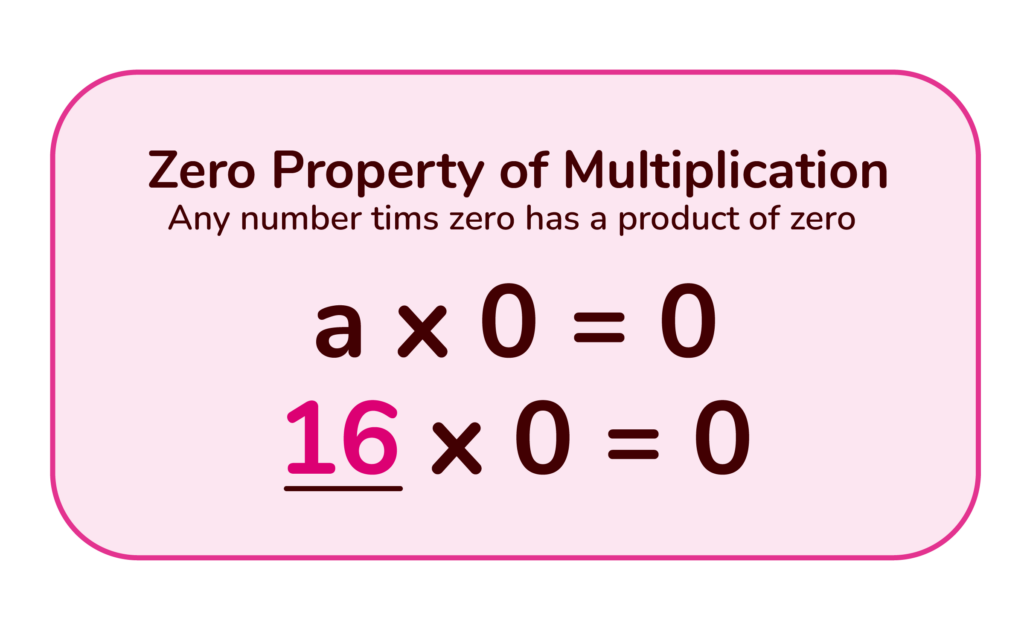
The zero property of multiplication states that any number multiplied by zero equals zero. For example, when we multiply 5 by 0 the outcome will always be 0.
This property is beneficial when solving equations or simplifying expressions, allowing us to “eliminate” terms multiplied by zero. It’s a fundamental rule in maths that provides a quick way to solve any multiplication problem where one of the factors is zero.
Unlock unlimited maths questions
Put your skills to the test with fun exercises + maths games that are proven to boost ability!
DoodleMaths is an award-winning app that’s filled with thousands of questions and games exploring multiplication, division and more!
Designed by teachers, it creates each child a unique work programme tailored to their needs, doubling their progression with just 10 minutes of use a day.* Try it for free!

*Based on earning 24 stars a day in DoodleMaths. Read full study

Apply the associative property of multiplication to simplify these problems:
Use the commutative property of multiplication to rewrite and solve these problems:
Use the distributive property of multiplication to simplify these problems:
Use the identity property of multiplication to solve these problems:
Apply the zero property of multiplication to solve these problems:
Associative property of multiplication
Commutative property of multiplication
Distributive property of multiplication
Identity property of multiplication
Zero property of multiplication
The five properties of multiplication are the Associative Property of Multiplication, Commutative Property of Multiplication, Identity Property of Multiplication, Distributive Property of Multiplication, and Zero Property of Multiplication.
Applying the properties of multiplication is all about recognising the conditions that allow each property to be used. For instance, if you see a problem that requires you to multiply a number by the sum of two other numbers, such as 2 * (3 + 4), you might recognise it as the distributive property.
The commutative property of multiplication means that the order of numbers in a multiplication problem doesn’t change the result. For instance, 5 x 3 = 3 x 5. Many consider it similar to the multiplication property of equality.

Remember, practising consistently is vital to mastering these properties. For additional practice, you can check out this maths help app. It’s an excellent tool for extra help and provides more exercises.

Parents, sign up for a DoodleMaths subscription and see your child become a maths wizard!

Lesson credits

Michelle Griczika
Michelle Griczika is a seasoned educator and experienced freelance writer. Her years teaching coupled with her double certification in early childhood education lend depth to her understanding of diverse learning stages. Michelle enjoys running in her free time and undertaking home projects.

Michelle Griczika
Michelle Griczika is a seasoned educator and experienced freelance writer. Her years teaching coupled with her double certification in early childhood education lend depth to her understanding of diverse learning stages. Michelle enjoys running in her free time and undertaking home projects.
Book a chat with our team
If you’d like to use Doodle’s browser version, please visit this page on a desktop.
To log in to Doodle on this device, you can do so through our apps. You can find out how to download them here: