They may look funky at first, but we promise mixed numbers and improper fractions don’t have to throw you for a loop – let’s learn about them together!
Author
Taylor Hartley
Expert Reviewer
Jill Padfield
Published: January 23, 2024
They may look funky at first, but we promise mixed numbers and improper fractions don’t have to throw you for a loop – let’s learn about them together!
Author
Taylor Hartley
Expert Reviewer
Jill Padfield
Published: January 23, 2024
They may look funky at first, but we promise mixed numbers and improper fractions don’t have to throw you for a loop – let’s learn about them together!
Author
Taylor Hartley
Expert Reviewer
Jill Padfield
Published: January 23, 2024
Key takeaways
Platform 9 ¾. The Lion King 1 ½. You’ve probably seen mixed numbers before, but you might not know exactly what they mean or how they’re created. Now, your teacher’s asking you to turn improper fractions into mixed numbers, mixed numbers into improper fractions, and mixed numbers into decimals – all at one time!
Don’t worry: mixed numbers aren’t nearly as wonky as they look. Today, we’ll take a look at what mixed numbers are, how to convert improper fractions into mixed numbers and vice versa, and what to do when your teacher asks, “Can you make that into a decimal for me?”. Let’s dive in.
We know what numbers are, right? And we know that when you “mix” things together, you’re bringing together two things that may be related, but are not entirely the same thing. So think of a “mixed number” as a combination of both a whole number and a fraction, or a part of a whole.
Let’s think about it another way. Say you’re having a pizza party. Your mum orders several pizzas so you and your friends can each have more than one slice. If your friends eat one whole pizza and then half of another pizza, you’ve eaten 1 ½ pizzas. That’s a mixed number!
Unlock unlimited maths questions
Put your learning into practice with fun exercises + games that are proven to boost ability!
The first part of your quotient – or the answer to Step 1 – becomes the whole number part of the mixed number: 2.
Your remainder, 2, becomes the numerator in the fraction part of your mixed number. Now, the denominator is still 6, because that’s how many “parts” are in the whole, or in our example, how many slices make a whole pizza.
Your answer is a mix of your whole number and your fraction (or how many pizzas you have and how many extra slices are leftover).
So 2 is the whole number. 2⁄6 is the fraction. And 2 goes into six 3 times, so you can simplify 2⁄6 to be ⅓.
Your mixed number is 2 ⅓.
Let’s do the reverse now. This is like counting up all the slices you have and saying how many slices you have over how many pizzas you can make.
Let’s say you have 1 ¾ of pizzas.
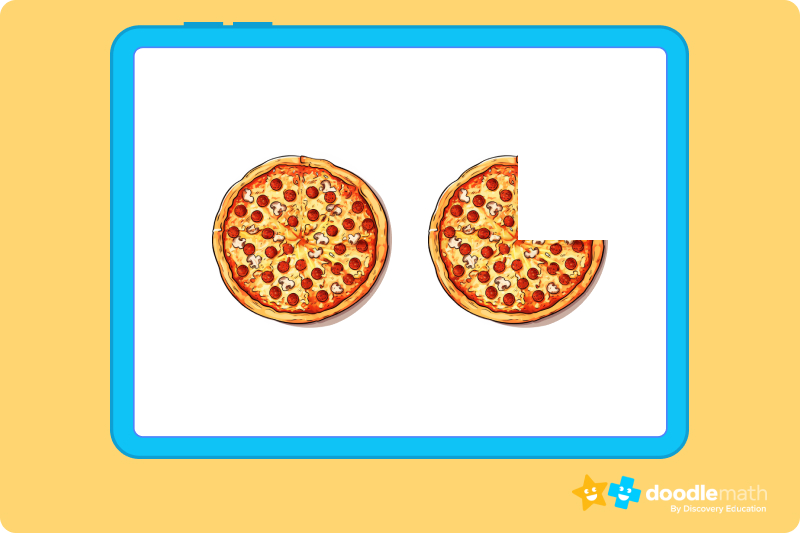
In our example, you multiply 1 (your whole number) by 4 (the fraction’s denominator). You get ‘4’.
You add 4 (the result from step 1) to 3 (the fraction’s numerator) and get ‘7’.
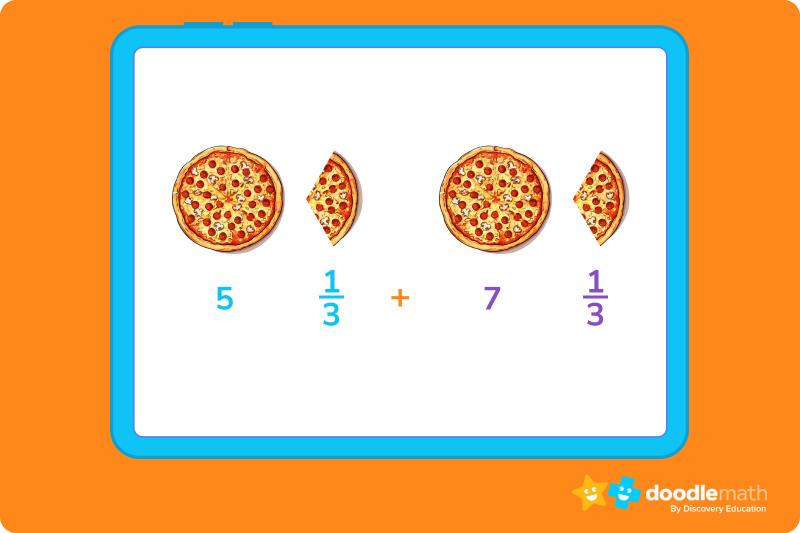
5 ⅓ + 7 ⅓ = ?
The fractions in each of these problems tell us that there are 3 pieces per pizza. First, let’s figure out how many individual slices we have on each table.
5 pizzas × 3 slices per piece = 15 pieces on the first table, along with the 1 slice from 1⁄3. That’s 16 slices.
On the second table, we have 7 pizzas with 3 slices each, so 7 pizzas × 3 slices = 21 slices, plus the 1 slice from ⅓, so 22 slices.
So, 5 ⅓ = 16⁄3 as an improper fraction.
And 7 ⅓ = 22⁄3 as an improper fraction.
Now we know that there are 16 slices on one table and 22 slices on the other. Since, all our slices are ⅓ of a pizza, so we can just add them together.
Numerators: 16 + 22 = 38
Improper fraction: 38⁄3
Meet DoodleMaths, the award-wining maths app that’s proven to double a child’s rate of progression with just 10 minutes of use a day!
Filled with fun, interactive questions covering the whole curriculum, it creates each child a unique work programme tailored to their needs, boosting their confidence and skills in maths. Try it free today!
The whole number is 3.
We do this by dividing the numerator (1) by the denominator (4)
Our decimal is 0.25
So 3 ¼ pizzas is the same as 3.25 pizzas in decimal form.
You’ve done a great job following along! Now it’s time for the best part – practice. The more we do it, the better we get! Remember, we’re going to treat each problem just like we’re figuring out our pizzas. Let’s get started!
Let’s go through it step by step.
1. Divide the numerator (7) by the denominator (3).
We know that 3 goes into 7 two times, so 7 ÷ 3 = 2 with a remainder of 1.
2. The whole number part of the mixed number is the quotient from step 1, which is 2.
Whole number: 2
3. The numerator of the fractional part is the remainder from step 1, which is 1.
Numerator: 1
4. The denominator remains the same.
Denominator: 3
Therefore, the mixed number representation of 7⁄3 is 2 1⁄3.
Let’s break it down:
1. Divide the numerator (10) by the denominator (4).
10 ÷ 4 = 2, with a remainder of 2.
2. The whole number part of the mixed number is the quotient from step 1, which is 2.
Whole number: 2
3. The numerator of the fractional part is the remainder from step 1, which is 2.
Numerator: 2
4. The denominator remains the same.
Denominator: 4
Therefore, the mixed number representation of 10⁄4 is 2 2⁄4, or if we simplify 2 2⁄4, it’s 2 ½.
Here we go again!
1. Divide the numerator (13) by the denominator (5).
13 ÷ 5 = 2 with a remainder of 3
2. The whole number part of the mixed number is the quotient from step 1, which is 2.
Whole number: 2
3. The numerator of the fractional part is the remainder from step 1, which is 3.
Numerator: 3
4. The denominator remains the same.
Denominator: 5
Therefore, the mixed number representation of 13⁄5 is 2 3⁄5.
Now we’re switching it up! Let’s use our steps to walk through this:
1. Start with the whole number part, which is 2. Write it down as a whole number.
Whole number: 2
2. Next, convert the fractional part to a decimal. Divide the numerator (3) by the denominator (4).
3 ÷ 4 = 0.75
3. Write down the decimal obtained from step 2.
Decimal: 0.75
4. Combine the whole number and the decimal obtained to get the final answer.
Final answer: 2.75
Therefore, the decimal representation of the mixed number 2 3⁄4 is 2.75.
Let’s practise together.
1. Change your mixed numbers into improper fractions.
For 1 ¾ Multiply the whole number (1) by your denominator (4) and add the numerator (3).
1×4+3= 7
Place your new numerator over your denominator to get 7⁄4
For 2 ½ multiply the whole number (2) by your denominator (2) and add the numerator (1).
2×2+1= 5
Place your new numerator over your denominator 5⁄2
2. Add your improper fractions.
To do this, find the common denominator for 7⁄4 and 5⁄2, which is 4. Rewrite both fractions with the common denominator.
Multiply the numerator and denominator in your second fraction (5⁄2) by 2 making 10⁄4. Now that the denominators are the same, add the numerators.
10⁄4 + 7⁄4 = 17⁄4
3. Convert your improper fraction to a mixed number.
First, divide the numerator (17) by the denominator (4) giving you a quotient of 4. This is your whole number. The remainder (1) is your new numerator.
The answer is 4 ¼.
Ready to give it a go?
Great job learning about fractions and mixed numbers. Now, let’s practise. Practising helps us get better and lets us show what we can do. Remember to tackle each problem just like we’ve learned. Let’s go!
Click to reveal the answer.
The answer is 4½.
The answer is 2.75.
The answer is 2.5.
The answer is 4.
The answer is 4½.
How did we get here?
1. Divide the numerator (9) by the denominator (2).
9 ÷ 2 = 4, with 1 left over (remainder).
2. The whole number part of the mixed number is the quotient from step 1, which is 4.
Whole number: 4
3. The numerator of the fraction is the remainder from step 1, which is 1.
Numerator: 1
4. The denominator remains the same.
Denominator: 2
Therefore, the improper fraction 9⁄2 is 4 ½ as a mixed number.
The answer is 21⁄4.
How did we get here?
1. Multiply the whole number (5) by the denominator of the fraction (4).
5 x 4 = 20
2. Add this result to the numerator of the fraction (1).
20 + 1 = 21
3. This sum becomes your new numerator, and the denominator stays the same.
Numerator: 21
Denominator: 4
So, the improper fraction representation of 5 1⁄4 is 21⁄4.
The answer is 2.75.
Here’s how we do it.
1. Keep the whole number part, which is 3.
Whole number: 3
2. Convert the fractional part (1⁄3) into a decimal by dividing the numerator (1) by the denominator (1). This gives you 0.33.
Decimal fraction: 0.33
3. Add 0.33 to 1, so you get 1.33.
So, 1 1⁄3 as a decimal is 1.33.
The answer is 2.5.
How did we get here?
1. Keep the whole number part, which is 2.
Whole number: 2.
2. Convert the fractional part (1⁄2) into a decimal by dividing the numerator (1) by the denominator (2). This gives you 0.5
Decimal fraction: 0.75.
3. Add 0.5 to 2, so you get 2.05.
So, 2 1⁄2 as a decimal is 2.05.
The answer is 4.
Let’s go through the process.
1. Convert these mixed numbers into improper fractions.
2 2⁄3 becomes 8⁄3 and 1 1⁄3 becomes 4⁄3.
2. Now, let’s add the fractions. Since the denominators are already the same (which is 3), we just add the numerators.
This gives us (8 + 4)/3, which simplifies to 12⁄3.
3. Lastly, let’s convert the resulting improper fraction back into a mixed number.
This gives you 4.
So, 2 2⁄3 + 1 1⁄3 = 4.
Sign up for the DoodleMaths app today!
Turn math into an adventure when you sign up for DoodleMaths.
Mixed numbers and improper fractions are different ways to write the same amount. In an improper fraction, the denominator tells us the size of the parts and the numerator tells us the number of parts. In a mixed number, the denominator tells us the size of the parts and the whole number and numerator in the fraction tell us the number of parts together.
First, multiply the whole number by the denominator. Next, add the numerator to this result. Then, write your new fraction with the answer from the second step. The denominator remains the same.
First, divide the numerator by the denominator. Next, use the result (quotient) as the whole number. Then, use the remainder as the numerator of the fraction. The denominator remains the same.
Parents, sign up for a DoodleMaths subscription and see your child become a maths wizard!
Lesson credits
Taylor Hartley
Taylor Hartley is an author and an English teacher. When she's not writing, you can find her on the rowing machine or lost in a good novel.
Jill Padfield
Jill Padfield has 7 years of experience teaching mathematics. She is currently working as a Business Analyst, working to improve services for Veterans while earning a masters degree in business administration.
Taylor Hartley
Taylor Hartley is an author and an English teacher. When she's not writing, you can find her on the rowing machine or lost in a good novel.
Jill Padfield
Jill Padfield has 7 years of experience teaching mathematics. She is currently working as a Business Analyst, working to improve services for Veterans while earning a masters degree in business administration.
All rights reserved.
Book a chat with our team
If you’d like to use Doodle’s browser version, please visit this page on a desktop.
To log in to Doodle on this device, you can do so through our apps. You can find out how to download them here:
